I went through a great deal of trouble to contest the idiosyncratic claim of a critic at TheSkepticalZone who said:
if you have 500 flips of a fair coin that all come up heads, given your qualification (“fair coin”), that is outcome is perfectly consistent with fair coins,
This critic (who goes by the handle “Eigenstate”) would probably keep singing the same tune if we were dealing with 500,000,000 fair coins. I said this was wrong, and KeithS disagreed and demanded I make a retraction. See his comments here in SSDD: a 22 sigma event is consistent with the physics of fair coins?.
I insisted the expectation value of 50% heads has to be respected, that if a theory predicts a certain expectation value, multi-sigma deviations from that expectation are reasonable grounds (not absolute grounds) to reject that theory as an explanation of that phenomenon. Yet KeithS keeps swearing by the fact (which I don’t dispute, nor never have disputed) that “all coins heads” is no more improbable than any other sequence. I never said otherwise the original post, Siding with Mathgrrl on a point, that generated the current firestorm of threads and comments.
But the fact that “all coins heads” is no more improbable than any other sequences does not negate the fact that all-coins heads is statistically inconsistent with the hypothesis of a fair coin and a random process acting on the fair coins. At issue is the fact all coins heads has a statistical property, namely, it is maximally deviant from the expectation value.
I never said “all heads coins” was more improbable than any other sequence, but I did say the following, that seemed to go in one ear and out the other over at TSZ:
For example, consider if we saw 500 fair coins all heads, do we actually have to consider human subjectivity when looking at the pattern and concluding it is designed? No. Why? We can make an alternative mathematical argument that says if coins are all heads they are sufficiently inconsistent with the Binomial Distribution for randomly tossed coins, hence we can reject the chance hypothesis.
They failed to acknowledge I used the Binomial Distribution. I then spelled it out for them what that meant in terms of standard deviations and expectation values in SSDD: a 22 sigma event is consistent with the physics of fair coins?.
But my critics were not merely content to hear me criticize some of Bill Dembski’s work, they wanted find fault where there was none, because the fact I might have a legitimate point is intolerable since creationists supposedly don’t like science and math.
Anti-ID critics have propensity to :
1. misread
2. misattribute
3. mischaracterize
4. misstate
5. render the most uncharitable interpretation of what is said
6. and when called on their uncharitable readings and errors, they compound their errors because of a determination to save face
Over at TSZ, I’ve happily offered statements, and then retracted errors in my calculations (see my post on the 2nd law and you’ll see I welcomed correction of my misunderstanding of the Liouville theorem). I’m grateful for the interaction with critics because:
1. they do correct errors
2. they do educate
3. they help us learn to state our points in ways less likely to be misread in the future
So, I do think the exchange is valuable. But well, in contrast, for people like KeithS, in an attempt to save face, they just say even more idiosyncratic things and keep demanding I make a retraction. I told KeithS: “No DICE”.
From Wiki on Law of Large Numbers:
In probability theory, the law of large numbers (LLN) is a theorem that describes the result of performing the same experiment a large number of times. According to the law, the average of the results obtained from a large number of trials should be close to the expected value, and will tend to become closer as more trials are performed.
The LLN is important because it “guarantees” stable long-term results for the averages of random events. For example, while a casino may lose money in a single spin of the roulette wheel, its earnings will tend towards a predictable percentage over a large number of spins. Any winning streak by a player will eventually be overcome by the parameters of the game. It is important to remember that the LLN only applies (as the name indicates) when a large number of observations are considered.
Say what? All the calculations I made regarding expectation values might actually be meaningful!
Also from Wiki:
For example, a single roll of a six-sided die produces one of the numbers 1, 2, 3, 4, 5, or 6, each with equal probability. Therefore, the expected value of a single die roll is
According to the law of large numbers, if a large number of six-sided dice are rolled, the average of their values (sometimes called the sample mean) is likely to be close to 3.5, with the accuracy increasing as more dice are rolled.
It follows from the law of large numbers that the empirical probability of success in a series of Bernoulli trials will converge to the theoretical probability. For a Bernoulli random variable, the expected value is the theoretical probability of success, and the average of n such variables (assuming they are independent and identically distributed (i.i.d.)) is precisely the relative frequency.
For example, a fair coin toss is a Bernoulli trial. When a fair coin is flipped once, the theoretical probability that the outcome will be heads is equal to 1/2. Therefore, according to the law of large numbers, the proportion of heads in a “large” number of coin flips “should be” roughly 1/2. In particular, the proportion of heads after n flips will almost surely converge to 1/2 as n approaches infinity.
Though the proportion of heads (and tails) approaches 1/2, almost surely the absolute (nominal) difference in the number of heads and tails will become large as the number of flips becomes large. That is, the probability that the absolute difference is a small number, approaches zero as the number of flips becomes large. Also, almost surely the ratio of the absolute difference to the number of flips will approach zero. Intuitively, expected absolute difference grows, but at a slower rate than the number of flips, as the number of flips grows.
No where in that wiki article is anything there that can be remotely construed to defend statements like:
if you have 500 flips of a fair coin that all come up heads, given your qualification (“fair coin”), that is outcome is perfectly consistent with fair coins,
Eigenstate criticizing Sal
Look at this graph of dice rolls and how large numbers of trials converge on expectation value of 3.5 (a similar feature will emerge with fair coins converging on expectation value of 0.5 heads). These are principles that KeithS and eigenstate don’t like discussing in their determined attempt to misread and disagree with everything I say, even textbook statistics. They will compound their errors because anti-ID critics have a propensity to never admit error, and will argue to save face at all costs, and this will lead to some very entertaining reading. 🙂
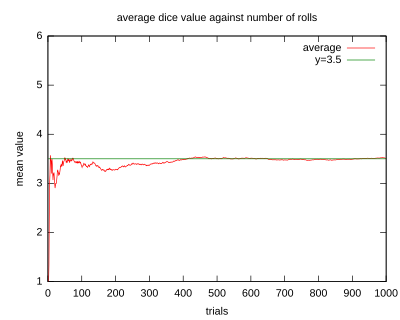
But alas, KeithS and others refuse to acknowledge these considerations, and worse he demands I make a retraction as if I’m some sort of mathematical heritic. No Dice, KeithS.
Same Silliness, Different Darwinist (SSDD).
ADDENDUM
At the request of Elizabeth Liddle, I’m highlighting her comment:
Look at the use of the word “consistent”. Eigenstate used it to mean “compatible with the stipulation that the coin is fair”. Sal interpreted it to mean “compatible with the conclusion that the coin is fair”.
Interpreted the first way, Eigenstate is correct. Interpreted the second way, Sal is correct.
I confess I don’t quite understand what Elizabeth means, but out of my great respect for her, I’m highlighting her comment.