The Online Dictionary’s Thesaurus tells us:
contradiction in terms – (logic) a statement that is necessarily false; “the statement `he is brave and he is not brave’ is a contradiction”
As a capital, classic example, say the following words:
“Square Circle”
Now, riddle me this, riddle me that, guess me this riddle and perhaps not:
DRAW ME ONE.
I confidently assert [HT: Peter Cech], this cannot be done:
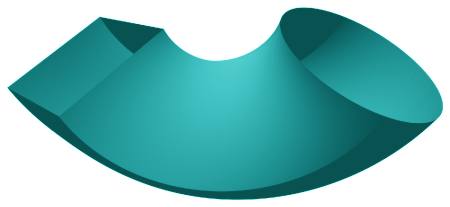
You will observe that the square and the circle show how a circle and a square can by degrees be transformed or mapped into each other, but that the one and the same object in the same place and time cannot have the essential properties of squareness and circularity.
In short, we here see how identity and non-contradiction are mutually reinforcing first principles of right reason (with also excluded middle involved). As Wikipedia, speaking against known general ideological interest affirmed as at c. Feb 2012, in an article on the laws of thought tracing to Dec 2004:
The law of non-contradiction and the law of excluded middle are not separate laws per se, but correlates of the law of identity. That is to say, they are two interdependent and complementary principles that inhere naturally (implicitly) within the law of identity, as its essential nature . . . whenever we ‘identify’ a thing as belonging to a certain class or instance of a class, we intellectually set that thing apart from all the other things in existence which are ‘not’ of that same class or instance of a class. In other words, the proposition, “A is A and A is not ~A” (law of identity) intellectually partitions a universe of discourse (the domain of all things) into exactly two subsets, A and ~A, and thus gives rise to a dichotomy. As with all dichotomies, A and ~A must then be ‘mutually exclusive’ and ‘jointly exhaustive’ with respect to that universe of discourse. In other words, ‘no one thing can simultaneously be a member of both A and ~A’ (law of non-contradiction), whilst ‘every single thing must be a member of either A or ~A’ (law of excluded middle).
What’s more . . . thinking entails the manipulation and amalgamation of simpler concepts in order to form more complex ones, and therefore, we must have a means of distinguishing these different concepts. It follows then that the first principle of language (law of identity) is also rightfully called the first principle of thought, and by extension, the first principle [of right] reason (rational thought) . . .
Why am I pounding away on such an obvious point?
Because it keeps cropping up in attempts to evade or dismiss the design inference, whether in the guise of denying non-contradiction [Cf. UD WAC here], or insisting on some imagined ambiguity or attempting to undermine some otherwise clear distinction.
So, let me raise again a fairly simple diagram:

If at a given moment we distinctly identify and label some thing, A — say, a bright red ball on a table — we mark a mental border-line and also necessarily identify NOT-A as “the rest of the World.” We thus have a definite separation of the World into two parts, and it immediately and undeniably holds that:
(a) the part labelled A will be A (symbolically, [A => A] = 1),
(b) A will not be the same as NOT-A ( [A AND NOT-A] = 0); and
(c) there is no third option to being A or NOT-A ( [A OR NOT-A] = 1).
So, we see how naturally the laws of (a) identity, (b) non-contradiction (or, non-confusion!), and (c) the excluded middle swing into action. This naturalness also extends to the world of statements that assert that something is true or false, as we may see from Aristotle’s classic remark in his Metaphysics 1011b (loading the 1933 English translation):
. . . if it is impossible at the same time to affirm and deny a thing truly, it is also impossible for contraries to apply to a thing at the same time; either both must apply in a modified sense, or one in a modified sense and the other absolutely.
Nor indeed can there be any intermediate between contrary statements, but of one thing we must either assert or deny one thing, whatever it may be. This will be plain if we first define truth and falsehood. To say that what is is not, or that what is not is, is false; but to say that what is is, and what is not is not, is true; and therefore also he who says that a thing is or is not will say either what is true or what is false. [Emphases added]
So, we can state the laws in more or less traditional terms:
[a] A thing, A, is what it is (the law of identity);
[b] A thing, A, cannot at once be and not-be (the law of non-contradiction);
[c] A thing, A, is or it is not, but not both or neither (the law of the excluded middle).
As a consequence of this, if we say something that directly asserts a contradiction in terms, or that implies a contradiction, that thing we have said is nonsense. We can make mouth noises or string glyphs that reduce to such, but we cannot instantiate what we think we have said on the ground, physically or even in some cases, logically.
The square circle — despite many valiant attempts — is such an entity.
Similarly, there is a classic (probably apocryphal) story of Abraham Lincoln:
A favorite riddle: “How many legs will a sheep have if you call the tail a leg?” Most people answered, “five.” Lincoln then replied that the answer was four, because calling a tail a leg doesn’t make it a leg. His moral was that you can’t solve a problem by simply changing a word or name.
In short, merely saying X does not make X true. “Saying does not make it so.”
I first heard of this in connexion with an aide, but here is a case (probably just as apocryphal) suggested, with a congressman:
The Great Emancipator, Abraham Lincoln, was asked by a congressman why he hadn’t freed the slaves earlier in his term of office.
Lincoln replied that the time had not been right; he wouldn’t have been able to enforce the proclamation. The congressman was puzzles and didn’t understand what the president meant.
Lincoln explained with a question, “How many legs will a sheep have, if you call the tail a leg?”
“Five,” responded the congressman.
“Not so,” said Lincoln wisely. “Calling a tail a leg doesn’t make it so.”
But in today’s age of radical relativism and spin tactics, we have a challenge to deal with the nihilism that imagines that might and manipulation make ‘right,’ ‘knowledge,’ and ‘truth.’
Which is outright nonsense.
Just so, and with all due respect, for one instance that has spilled more bits than it is worth here at UD recently, playing games on suggesting that there is a distinction between Intelligent Design and intelligent design, such that the former represents a grand conspiracy that is a hidden agenda theocratic wedge while the latter (it is hard to be sure) seems to suggest that recognising design on empirically observable reliable signs is not possible, does not answer to the question of the existence of and grounds for design inference and its proper grounding, as can be seen again in the per aspect design filter summary:

I recently (again) summarised the fundamental question that design theory as a scientific project addresses here, in a comment:
1 –> Yes, we can find objects that credibly exhibit traces of the remote past of origins, a far or deep past that we cannot DIRECTLY observe to know by that, the actual deep past as empirical fact.
2 –> However, this is not unique to this case, there are many objects, such as astronomical ones, that we study be observing traces, e,g. light from the sun and remote stars.
4 –> In this general context, Newton put forth his well known rules of reasoning c 1688 — 1704, and it is the context in which, generally, we infer through cause-effect reasoning and characteristic consequences of particular causes. Such can be identified as signs.
5 –> We then provisionally but confidently infer on signs, per like causes like. For simple example, we see that certain elements, when hot enough, give off certain spectral lines, and that when white light passes through layers of such, there are absorption lines in the relevant positions. From these, we examine the spectra of stars and infer composition from Fraunhoffer lines. (Sometimes this has led to interesting results, e,g. discovery of Helium as causing unexpected lines in the sun’s spectrum.)
6 –> This basic pattern is commonly applied to origins of life and of forms, though this is often marred by a want of observational evidence that certain claimed causes are adequate to claimed effects, especially on OOL and OO body plans. Johnson, whom you deride, has aptly pointed out the injection of an ideological materialist a priori, in the guise of mere “reasonable” methodological constraints. Lewontin’s case is most notorious and explicit, but cf here on for much more.
7 –> In the case of functionally specific, complex information and irreducible complexity, these are commonly observed phenomena. In comparing causes dominated by mechanical necessity manifest in lawlike regularities like F = m*a, chance processes yielding statistical variability, and design as known causal factors, it is seen first that necessity does not explain high contingency under similar initial conditions. Indeed, that lack is the sign of a law at work.
8 –> Similarly, high contingency tracing to blind chance, per the results of sampling theory, is at a loss to explain results coming from specific and separately describable UN-representative zones in the field of possibilities. That is, a sample that is necessarily a small grab of a very large space, we can only expect to reflect the BULK of the possibilities. This is how, for instance, we infer to the general pattern of the blood from a small sample.
9 –> We can also show that where we have specific function depending on many well matched, properly arranged and interfaced parts, the zone of functional configs will be a very small fraction of the space of possibilities for the parts. Not only do tornadoes predictably fail to assemble 747′s from junkyard parts, but they will fail to do so for something so deceptively simple as a moving coil meter based indicating instrument in its cockpit.
10 –> This is the context in which on empirical and analytical grounds — once ideological blinkers are removed — it is clear that functionally specific, complex organisation and/or associated information [FSCO/I] are a strong sign of design as best causal explanation.
11 –> To set a conservative threshold for sufficient complexity, note that 500 bits of explicit or implied information implies 3.27*10^150 possibilities. The 10^57 atoms of our solar system, on its conventionally estimated age, if used to search at one search per 10^-14 s [comparable to the fastest chemical reaction rates] would only be able to sample what we can compare as taking a one straw sized sample to a cubical hay bale as thick as our galaxy, about 1,000 light years. (Light from that far away reaching us now, set out sometime about the time of the Norman Conquest of England.)
12 –> So, we have strong reason indeed to accept that if Chi_500 is at least 1, the object with that much specific info in it, was designed:
Chi_500 = I*S – 500, bits beyond the solar system threshold.
So, motive mongering and games with words are not relevant. The pivotal issue is, as always, the matter on the merits. Let us therefore [re-]turn to that. END