Dr Carol Wood of Wesleyan University (a student of Abraham Robinson who pioneered non-standard analysis 50+ years ago) has discussed the hyperreals in two Numberphile videos:
Wenmackers may also be helpful:
In effect, using Model Theory (thus a fair amount of protective hedging!) or other approaches, one may propose an “extension” of the Naturals and the Reals, often N* or R* — but we will use *N and *R as that is more conveniently “hyper-“.
Such a new logic model world — the hyperreals — gives us a way to handle transfinites in a way that is intimately connected to the Reals (with Naturals as regular “mileposts”). As one effect, we here circumvent the question, are there infinitely many naturals, all of which are finite by extending the zone. So now, which version of the counting numbers are we “really” speaking of, N or *N? In the latter, we may freely discuss a K that exceeds any particular k in N reached stepwise from 0. That is, some k = 1 + 1 + . . . 1, k times added and is followed by k+1 etc.
And BTW, the question of what a countable infinite means thus surfaces: beyond any finite bound. That is, for any finite k we represent in N, no matter how large, we may succeed it, k+1, k+2 etc, and in effect we may shift a tape starting 0,1,2 etc up to k, k+1, k+2 etc and see that the two “tapes” continue without end in 1:1 correspondence:
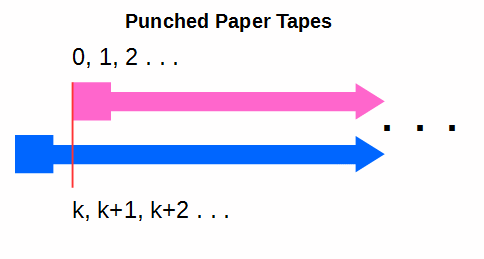
Extending, in a quasi-Peano universe, we can identify some K > any n in N approached by stepwise progression from 0. Where of course Q and R are interwoven with N, giving us the familiar reals as a continuum. What we will do is summarise how we may extend the idea of a continuum in interesting and useful ways — especially once we get to infinitesimals.
Clipping from the videos:

Pausing, let us refresh our memory on the structure and meaning of N considered as ordinals, courtesy the von Neumann construction:
- {} –> 0
- {0} –> 1
- {0,1} –> 2
- . . .
- {0,1,2 . . . } –> w, omega the first transfinite ordinal
Popping over to the surreals for a moment, we may see how to build a whole interconnected world of numbers great and small (and yes the hyperreals fit in there . . . from Ehrlich, as Wiki summarises: “[i]t has also been shown (in Von Neumann–Bernays–Gödel set theory) that the maximal class hyperreal field is isomorphic to the maximal class surreal field”):
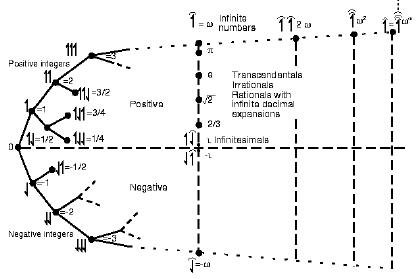
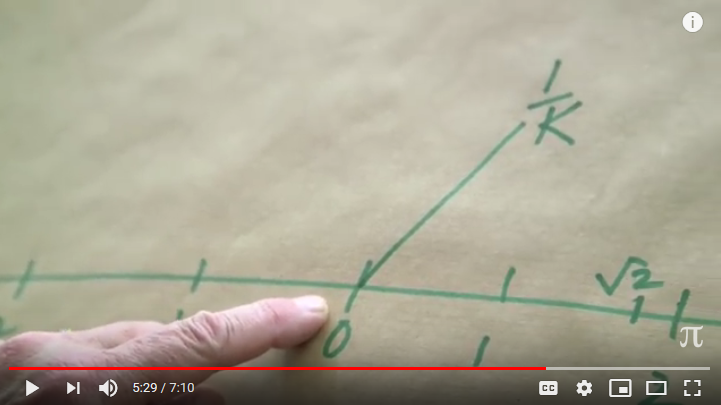
Now, we may also see in the extended R, *R, that 1/K = m, a newly minted number closer to zero than we can get by inverting any k in N or r in R, that is m is less than 1/k for any k in N and is less than 1/r for any r in R (as the reals are interwoven with the naturals):
and that as we have a range around K, K+1 etc and K-1 etc, even K/2 (an integer if K is even) m has company, forming an ultra-near cloud of similar infinitesimals in the extra-near neighbourhood of 0.
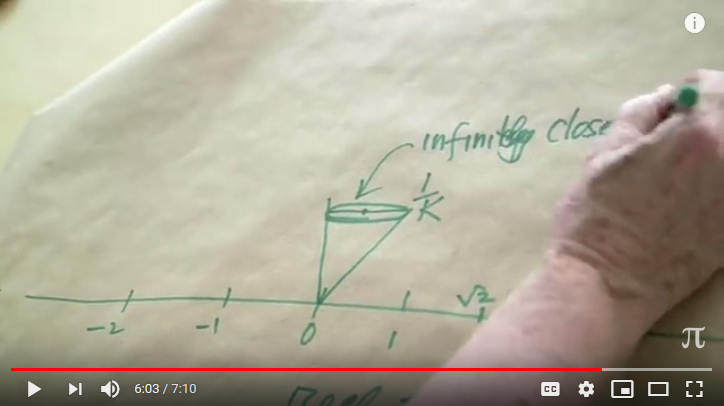
Of course, the reciprocal function is here serving as a catapult, capable of leaping over the bulk of the reals in *R, back and forth between transfinite hyperreals such as K and kin and infinitesimals such as m and kin, ultra-near to 0.
Using the additive inverse approach, this extends to the negative side also.
Further, by extending addition, 0 plus its cloud (often called a Monad — we can represent *0*) can be added to any r in R or indeed K in *R, i.e. we may vector-shift the cloud near 0 anywhere in *R. That is, every number on the extended line has its own ultra-near cloud. We note, reals are vectors with +/- directionality and a magnitude |r|.
Where does all of this lead? First, to Calculus foundations, then to implications of a transfinite zone with stepwise succession, among other things; besides, we need concept space to think and reason about matters of relevance to ID, however remotely (or not so remotely). So, let me now clip a comment I made in the ongoing calculus notation thread of discussion:
KF, 86: >>Money shot comment by JB:
JB, 74: we have Arbogast’s D() notation that we could use, but we don’t. Why not? Because we want people to look at this like a fraction. If we didn’t, there are a ton of other ways to write the derivative. That we do it as a fraction is hugely suggestive, especially, as I mentioned, there exists a correct way to write it as a fraction.
This is pivotal: WHY do we want that ratio, that fraction?
WHY do we think in terms of a function y = f(x), which is continuous and “smooth” in a relevant domain, then for some h that somehow trends to 0 but never quite gets there — we cannot divide by zero — then evaluate:dy/dx is
lim h –> 0
of
[f(x + h) – f(x)]/[(x + h) – x]. . . save that, we are looking at the tangent value for the angle the tangent-line of the f(x) curve makes with the horizontal, taken as itself a function of x, f'(x) in Newton’s fluxion notation.
We may then consider f-prime, f'(x) as itself a function and seek its tangent-angle behaviour, getting to f”(x), the second order flow function. Then onwards.
But in all of this, we are spewing forth a veritable spate of infinitesimals and higher order infinitesimals, thus we need something that allows us to responsibly and reliably conceive of and handle them.
I suspect, the epsilon delta limits concept is more of a kludge work-around than we like to admit, a scaffolding that keeps us on safe grounds among the reals. After all, isn’t there no one closest real to any given real, i.e. there is a continuum
But then, is that not in turn something that implies infinitesimal, all but zero differences? Thus, numbers that are all but zero different from zero itself considered as a real? Or, should we be going all vector and considering a ring of the close in C?
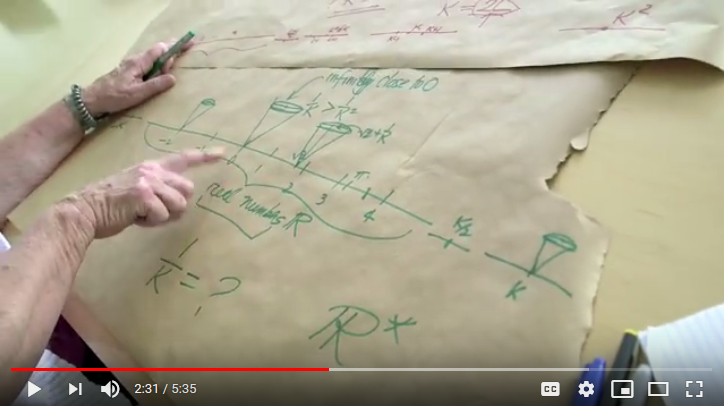
In that context, I can see that it makes sense to consider some K that somehow “continues on” from the finite specific reals we can represent, let’s use lower case k, and confine ourselves to the counting numbers as mileposts on the line:
0 – 1 – 2 . . . k – k+1 – k+2 – . . . . – K – K+1 – K+2 . . .
{I used the four dot ellipsis to indicate specifically transfinite span}
We may then postulate a catapult function so 1/K –> m, where m is closer to 0 than ANY finite real or natural we can represent by any k can give.
Notice, K is preceded by a dash, meaning there is a continuum back to say K/2 (–> considering K as even) and beyond, descending and passing mileposts as we go:K-> K-1 –> K-2 . . . K/2 – [K/2 – 1] etc,
but we cannot in finite successive steps bridge down to k thence to 1 and 0.
Where, of course, we can reflect in the 0 point, through posing additive inverses and we may do the rotation i*[k] to get the complex span.
Of course, all of this is to be hedged about with the usual non standard restrictions, but here is a rough first pass look at the hyperreals, with catapult between the transfinite and the infinitesimals that are all but zero. Where the latter clearly have a hierarchy such that m^2 is far closer to 0 than m.
And, this is also very close to the surreals pincer game, where after w steps we can constrict a continuum through in effect implying that a real is a power series sum that converges to a particular value, pi or e etc. then, go beyond, we are already in the domain of supertasks so just continue the logic to the transfinitely large domain, ending up with that grand class.
Coming back, DS we are here revisiting issues of three years past was it: step along mile posts back to the singularity as the zeroth stage, then beyond as conceived as a quasi-physical temporal causal domain with prior stages giving rise to successors. We may succeed in finite steps from any finitely remote -k to -1 to 0 and to some now n, but we have no warrant for descent from some [transefinite] hyperreal remote past stage – K as the descent in finite steps, unit steps, from there will never span to -k. That is, there is no warrant for a proposed transfinite quasi-physical, causal-temporal successive past of our observed cosmos and its causal antecedents.
Going back to the focus, if 0 is surrounded by an infinitesimal cloud closer than any k in R can give by taking 1/k, but which we may attain to by taking 1/K in *R, the hyperreals, then by simple vector transfer along the line, any real, r, will be similarly surrounded by such a cloud. For, (r + m) is in the extended continuum, but is closer than any (r + 1/k) can give where k is in R.
The concept, continuum is strange indeed, stranger than we can conceive of.
So, now, we may come back up to ponder the derivative.
If a valid, all but zero number or quantity exists, then — I am here exploring the logic of structure and quantity, I am not decreeing some imagined absolute conclusion as though I were omniscient and free of possibility of error — we may conceive of taking a ratio of two such quantities, called dy and dx, where this further implies an operation of approach to zero increment. The ratio dy/dx then is much as conceived and h = [(x +h) – x] is numerically dx.
But dx is at the same time a matter of an operation of difference as difference trends to zero, so it is not conceptually identical
Going to the numerator, with f(x), the difference dy is again an operation but is constrained by being bound to x, we must take the increment h in x to identify the increment in f(x), i.e. the functional relationship is thus bound into the expression. This is not a free procedure.
Going to a yet higher operation, we have now identified that a flow-function f ‘(x) is bound to the function f(x) and to x, all playing continuum games as we move in and out by some infinitesimal order increment h as h trends to zero. Obviously, f ‘(x) and f ”(x) can and do take definite values as f(x) also does, when x varies. So, we see operations as one aspect and we see functions as another, all bound together.
And of course the D-notation as extended also allows us to remember that operations accept pre-image functions and yield image functions. Down that road lies a different perspective on arithmetical, algebraic, analytical and many other operations including of course the vector-differential operations and energy-potential operations [Hamiltonian] that are so powerful in electromagnetism, fluid dynamics, q-mech etc.
Coming back, JB seems to be suggesting, that under x, y and other quasi-spatial variables lies another, tied to the temporal-causal domain, time. Classically, viewed as flowing somehow uniformly at a steady rate accessible all at once everywhere. dt/dt = 1 by definition. From this, we may conceive of a state space trajectory for some entity of interest p, p(x,y,z . . . t). At any given locus in the domain, we have a state and as t varies there is a trajectory. x and y etc are now dependent.
This brings out the force of JB’s onward remark to H:
if x *is* the independent variable, and there is no possibility of x being dependent on something else, then d^2x (i.e., d(d(x))) IS zero
Our simple picture breaks if x is no longer lord of all it surveys.
Ooooopsie . . .
Trouble.
As, going further, we now must reckon with spacetime and with warped spacetime due to presence of massive objects, indeed up to outright tearing the fabric at the event horizon of a black hole. Spacetime is complicated.
A space variable is now locked into a cluster of very hairy issues, with a classical limiting case. >>
The world just got a lot more complicated than hitherto we may have thought. END
F/N: I add (Apr 15) a link (HT: Wiki) on Faa di Bruno’s formula for the nth order derivative of a function of a function, which extends the well-known chain rule:

Hat tip MathWorld, here are the first few results:
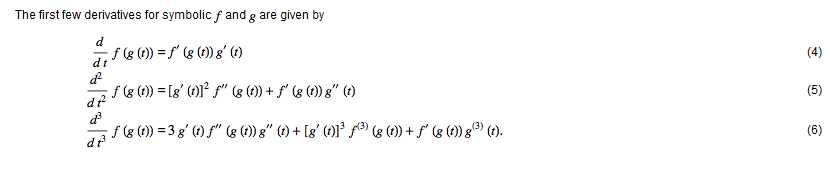
NB, this is referenced by JB in his discussion.
PS: As one implication, let us go to Davies and Walker:
In physics, particularly in statistical mechanics, we base many of our calculations on the assumption of metric transitivity, which asserts that a system’s trajectory will eventually [–> given “enough time and search resources”] explore the entirety of its state space – thus everything that is phys-ically possible will eventually happen. It should then be trivially true that one could choose an arbitrary “final state” (e.g., a living organism) and “explain” it by evolving the system backwards in time choosing an appropriate state at some ’start’ time t_0 (fine-tuning the initial state). In the case of a chaotic system the initial state must be specified to arbitrarily high precision. But this account amounts to no more than saying that the world is as it is because it was as it was, and our current narrative therefore scarcely constitutes an explanation in the true scientific sense. We are left in a bit of a conundrum with respect to the problem of specifying the initial conditions necessary to explain our world. A key point is that if we require specialness in our initial state (such that we observe the current state of the world and not any other state) metric transitivity cannot hold true, as it blurs any dependency on initial conditions – that is, it makes little sense for us to single out any particular state as special by calling it the ’initial’ state. If we instead relax the assumption of metric transitivity (which seems more realistic for many real world physical systems – including life), then our phase space will consist of isolated pocket regions and it is not necessarily possible to get to any other physically possible state (see e.g. Fig. 1 for a cellular automata example).
[–> or, there may not be “enough” time and/or resources for the relevant exploration, i.e. we see the 500 – 1,000 bit complexity threshold at work vs 10^57 – 10^80 atoms with fast rxn rates at about 10^-13 to 10^-15 s leading to inability to explore more than a vanishingly small fraction on the gamut of Sol system or observed cosmos . . . the only actually, credibly observed cosmos]
Thus the initial state must be tuned to be in the region of phase space in which we find ourselves [–> notice, fine tuning], and there are regions of the configuration space our physical universe would be excluded from accessing, even if those states may be equally consistent and permissible under the microscopic laws of physics (starting from a different initial state). Thus according to the standard picture, we require special initial conditions to explain the complexity of the world, but also have a sense that we should not be on a particularly special trajectory to get here (or anywhere else) as it would be a sign of fine–tuning of the initial conditions. [ –> notice, the “loading”] Stated most simply, a potential problem with the way we currently formulate physics is that you can’t necessarily get everywhere from anywhere (see Walker [31] for discussion).
[“The “Hard Problem” of Life,” June 23, 2016, a discussion by Sara Imari Walker and Paul C.W. Davies at Arxiv.]
Yes, cosmological fine-tuning lurks under these considerations, given where statistical mechanics points.
F/N: J P Moreland comments:

U/D April, 2022: As this topic was brought back up three years later, I add as follows:
KF, 221: >> . . .
I think some basics of structure and quantity need to be made explicitly clear. So, I note as below.
First, von Neumann’s construction:
{} –> 0
{0} –> 1
{0,1} –> 2
. . . [ellipsis]
{0,1,2 . . . } –> w
That is w [omega] is the order type of the naturals constructed as ordinals, and N has cardinality, aleph-null.
Now, let us do a comma separated value construction of a wx3 matrix, pardon limitations of Word Press:
{0: , 0 –>1 , 1 –> -1
1: , 2 –> 2 , 3 –> -2
2: , 4 –> 4, 5 –> -3
. . . , . . . , . . .
w: , w , w’}
Where clearly w + w’ = 0, as can be seen by decimation of the rows.
We here see next, that the naturals, the integers, the evens and the odds as well as the negatives all have order type w. Where, a basic definition of a transfinite set is that a proper subset can be placed in 1:1 correspondence with the whole. Thus, [by demonstration] the integers and particularly the negative integers can be seen to be transfinite, with the counting numbers as a metric. [Order type is w.]
Therefore, to claim that past finite stages of time that cumulate to now are without beginning, thus can be mapped to the set of the negative integers exhaustively, is to imply that the traverse from the remote pass to now is transfinite. Implicitly, transfinite.
That such a span cannot be traversed in cumulative, finite stage steps should be obvious and should be acknowledged. However, given the open or veiled acerbity that has generated a toxic climate surrounding origins in general and UD in particular, let us note for the simple case of counting onward from some k and its complement k’, again using a matrix:
0: , k+0 , k’-0
1: , k+1 , k’-1
2: , k+2 , k’-2
. . . , . . . , . . .
w: , k+w , k’ – w}
That is, counting on beyond any k we state or represent in N, with k’ in Z-, will continue transfinitely of order type w, just as for the col 1 case k = 0. This of course reflects the same transfiniteness. It also means that at any finite stage k, or k’ we may go on from there as though we had just begun.
A labour of Sisyphus.
This is how the futile supertask I have often spoken of arises.
And if counting in Z- L-ward, counting down is transfinite, the same members will be just as transfinite were we to start somehow from L-ward and try to proceed in R-ward steps to 0 and beyond. [Or even to some finite k’.]
We may therefore freely conclude that past time cannot have been transfinite, regardless of side debates as to how the difference between any two specific integers in Z we identify, state or represent, z1 and z2, will be finite as the two can be bounded by finite onward values. That is, the ellipsis is part of the structure of such sets and in part tells us that we can only execute stepwise a potential but not complete transfinite, and we use the ellipses to represent the actual main body of work, where the transfinite lives.
So, again, using hyperreals only emphasises and makes this plainer for someone first seeing such a strange domain.
We are only warranted to speak of a finitely remote past that has succeeded itself by finite stages to now. In principle, we may succeed onward in a potential infinity but at no particular stage will we exhaust such.>>